Distance (graph theory)
In the mathematical field of graph theory, the distance between two vertices in a graph is the number of edges in a shortest path connecting them. This is also known as the geodesic distance [1] because it is the length of the graph geodesic between those two vertices.[2] If there is no path connecting the two vertices, i.e., if they belong to different connected components, then conventionally the distance is defined as infinite.
The vertex set (of an undirected graph) and the distance function form a metric space, if and only if the graph is connected.
A metric defined over a set of points in terms of distances in a graph defined over the set is called a graph metric.
There are a number of other measurements defined in terms of distance:
The eccentricity 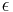 of a vertex
of a vertex 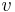 is the greatest geodesic distance between
is the greatest geodesic distance between 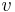 and any other vertex. It can be thought of as how far a node is from the node most distant from it in the graph.
and any other vertex. It can be thought of as how far a node is from the node most distant from it in the graph.
The radius of a graph is the minimum eccentricity of any vertex.
The diameter of a graph is the maximum eccentricity of any vertex in the graph. That is, it is the greatest distance between any pair of vertices. To find the diameter of a graph, first find the shortest path between each pair of vertices. The greatest length of any of these paths is the diameter of the graph.
A central vertex in a graph of radius  is one whose eccentricity is
is one whose eccentricity is  —that is, a vertex that achieves the radius.
—that is, a vertex that achieves the radius.
A peripheral vertex in a graph of diameter 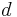 is one that is distance
is one that is distance 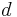 from some other vertex—that is, a vertex that achieves the diameter.
from some other vertex—that is, a vertex that achieves the diameter.
A pseudo-peripheral vertex 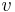 has the property that for any vertex
has the property that for any vertex  , if
, if 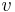 is as far away from
is as far away from  as possible, then
as possible, then  is as far away from
is as far away from 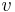 as possible. Formally, a vertex u is pseudo-peripheral, if for each vertex v with
as possible. Formally, a vertex u is pseudo-peripheral, if for each vertex v with 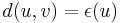 holds
holds  .
.
Algorithm for finding pseudo-peripheral vertices
Often peripheral sparse matrix algorithms need a starting vertex with a high eccentricity. A peripheral vertex would be perfect, but is often hard to calculate. In most circumstances a pseudo-peripheral vertex can be used. A pseudo-peripheral vertex can easily be found with the following algorithm:
- Choose a vertex
 .
. - Among all the vertices that are as far from
 as possible, let
as possible, let 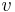 be one with minimal degree.
be one with minimal degree. - If
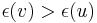 then set
then set 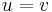 and repeat with step 2, else
and repeat with step 2, else 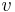 is a pseudo-peripheral vertex.
is a pseudo-peripheral vertex.
See also
- Distance matrix
- Resistance distance
- Betweenness
- Centrality
- Closeness
- Degree diameter problem for graphs and digraphs
Notes
- ^ Bouttier, Jérémie; Di Francesco,P. ,Guitter, E. (July 2003). "Geodesic distance in planar graphs". Nuclear Physics B 663 (3): 535–567. doi:10.1016/S0550-3213(03)00355-9. http://www.sciencedirect.com/science?_ob=ArticleURL&_udi=B6TVC-48KW72R-1&_user=3742306&_rdoc=1&_fmt=&_orig=search&_sort=d&view=c&_acct=C000061256&_version=1&_urlVersion=0&_userid=3742306&md5=86dd4de63373a7e72d23d16840947661. Retrieved 2008-04-23. "By distance we mean here geodesic distance along the graph, namely the length of any shortest path between say two given faces"
- ^ Weisstein, Eric W.. "Graph Geodesic". MathWorld--A Wolfram Web Resource. Wolfram Research. http://mathworld.wolfram.com/GraphGeodesic.html. Retrieved 2008-04-23. "The length of the graph geodesic between these points d(u,v) is called the graph distance between u and v"